Introduction
As any GPS software requires background maps in order to see where the action takes place, thus any raster image i.e. JPG or BMP file can be used as the background. However, this requires some calibration in order to have the map fitting its true position.
What Is Required?
You need a *.jpg (or *.jpeg) picture file (from a map or from a satellite picture). For this purpose, you can scan a map, or make a screenshot from, for example, GoogleMap. You also need some reference points in order to calibrate it.
Moreover, you need to determine in which hemisphere you live in, i.e. Northern or Southern and Eastern or Western. The solution might change if you are in a different hemisphere. Since we are in N/E we'll give the solution that fits our hemisphere.
Explanations
As you might already know, formats such as BMP, JPG, TIFF, etc. are called Raster Images. The reason to this is the means by which information is stored in their structures, Pixels. In other words the top left corner of the picture is (0,0) pixel i.e. its x=0 increases in the positive X axis (from left to right) and y=0 increases in the negative Y axis (from top to bottom).
In the N/E hemisphere (our hemisphere), Longitude increases in the positive x direction (left to right) and the Latitude increases in the positive y direction (bottom to top*).
* Note the direction of y has changed here.

Now calibrating the map means transferring from x,y coordinate system to longitude, latitude coordinate system or vice versa. This purpose can be achieved in several ways. The method we chose to go with is calculating the coordinate of the top left corner and the rate of change in both latitude and longitude.
Code Clarification
So we need to define some relationship between the two coordinate systems and the simplest method is knowing the X,Y as well as Lat, Long coordinates of one point (In this case, we take 0,0 pixel i.e. Top Left Corner). Ideally the Latitude along the X-axis should not increase if we keep y=0. For example if point (0,0) has Latitude of 10 degrees then all points in the x direction should have lat = 10 degrees. Similarly the Y-axis should obey the mentioned rule.
Rate In Each Axis
Let’s take a look at another new concept, rate in each axis. If we move 1 pixel in Y direction how many degrees/Pixels does this represent?
We know three points for which the latitude and longitude are available. So what we do is take the point with the minimum longitude (leftmost point) and the corresponding x pixel. Name this Long1 and X1.
Next, take the point with the maximum longitude (rightmost point) and the matching x pixel. Name this Long2 and X2.
Calculate the rate of change in X direction, delX as:
| [1] degrees per pixel in x direction |
delX= (Long2-Long1)/ (X2-X1) |
delX = (maxLONG().LONG - minLONG().LONG) / (maxLONG().X - minLONG().X);
Similarly, take the maximum latitude (topmost point) and the corresponding y pixel. Name it Lat1 and Y1, the minimum latitude and the matching y pixel, Lat2 and Y2. We calculate delY as:
| [2] degrees per pixel in y direction |
delY= (Lat2-Lat1)/ (Y2-Y1) |
delY = (minLAT().LAT - maxLAT().LAT) / (minLAT().Y - maxLAT().Y);
Now we're on with the calculation of the reference point (Top, Left). Note the maximum latitude (uppermost point) as Lat4 and Y4.
| [3] reference latitude |
lat0= Lat4+y4 * delY |
lat0 = maxLAT().LAT - maxLAT().Y * dY;
Also the minimum longitude (leftmost point) Long1, X1:
| [4] reference longitude |
long0= Long1 -x1 * delX |
long0 = minLONG().LONG - minLONG().X * dX;
Lat0 and Long0 are the geo coordinate representation of point (0,0).
Having all the above information and formulas, we can simply use the following equations to calculate the X and Y points of any given Latitude and longitude.
Conversion between Geographic and Pixel Coordinates
To convert from latitude and longitude to X and Y, you use the following equation:
| [5a – 6a] X and Y Coordinates |
X= (long-long0)/delx
Y= (lat-lat0)/delY |
public point LatLongtoXY(float Lat, float Long)
{
float X = (Long - LONG0) / dX;
float Y = (Lat - LAT0) / dY;
point pnt = new point(X, Y, Lat, Long);
return pnt;
}
To convert to latitude and longitude from any X and Y point:
| [5b - 6b] X and Y Coordinates |
Longitude= long0 + delX * X
Latitude=lat0 + delY * Y |
public point XYtoLatLong(float X, float Y)
{
float Lat = LAT0 + dY * Y;
float Long = LONG0 + dX * X;
point pnt = new point(X, Y, Lat, Long);
return pnt;
}
As a result, equations [1 2 3 4] will only be used once to digitize (Georeference) the map. From there, you only need equations [5a 5b – 6a 6b] to handle the conversions.
Using the Code
In order for the code to work, you need to build the geoReference library and use it in your projects.
geoReference contains a class point, which represents x, y, latitude and longitude of the reference points. We first create an array of points that hold the three reference points:
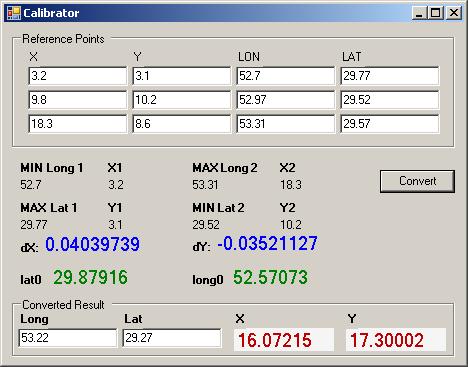
geoReference.point[] p = new geoReference.point[]
{
new geoReference.point(3.2f,3.1f,29.77f,52.7f),
new geoReference.point(9.8f,10.2f,29.52f,52.97f),
new geoReference.point(18.3f,8.6f,29.57f,53.31f)
};
Then we use the geoReference’s methods to convert from x,y to lat/long and vice versa:
geoReference gr = new geoReference(p);
geoReference.point pt = gr.LatLongtoXY(29.27f, 53.22f);
float x = pt.X;
float y = pt.Y;
Conclusion
You could now load a raster map in a picture box and use the above code to calibrate it. As an outcome if you pass the x and y coordinates to the mouse move event you could see the corresponding geo coordinates.
Special thanks …
To Diego Mijelshon for his excellent article ObjectComparer. It helped us with part of the code implementation.
History
- 5th February, 2006: Initial post
